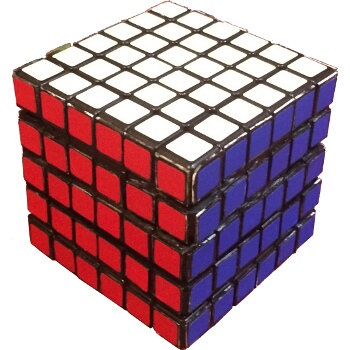
The world first fully functional (and in some regard proportional) 5x6x6 cuboid.

• Inventor: Ilya Toporgilka
• Mechanism: LxMxN (other)
• Patents: Unknown
• Producer: Custom
• Year: 2016
• Original Price: $0.00 USD
• Current Price: No Data

The inventor made this puzzle out of a massproduced 6x6x6. Although the cubies on this puzzle are proportional, the inventor could not find a way to have no gaps between them, like on conventional proportional cuboids.

After a few years after making the puzzle Ilya Toporgilka heard from Traiphum that his 2x4x5 cuboid had 18x18, 18x21 and 21x21 squares on 2 4x5 sides. Before this he had no idea that those cubies, in fact, have this difference. This made him think that 20% add on on the squares cannot be noticed. And his aim was to make puzzles that are "almost" proportional which means "a little disproportional", but unnoticeable. He refered to molecules and atoms or even something bigger like bacteria. He noticed even in his first school form that 2-3 or a little more spoons, as it seems, do not make the volume of the tea in the cup less. In the beginnings it cannot be noticed. Not to forget the water in the cup that can curve 1-2 mm. With a 6x6x6 each cubie is 11 mm. It means 2,2 mm can be added, and on 5x6 sides there will be 13.2 mm cubies. This number multiplied by 5 gives 66. This result also gets after 6 being multiplied by eleven. So, his conclusion was that his 5x6x6 looks like it is proportional. And the only way to check that it "lies" was only to measure each piece and edge lengths. He was trying to achieve some sort of "proportional puzzle mock up", though some of his puzzles like that even still look like they are proportional even after scrambling. He built then dozens of puzzles like that. With this 5x6x6 he decided to save some time and not fisher modify the cubies, but just leave the gaps(cutting just 2 mm from a layer can be a nightmare).
In 2018 Traiphum Pruntaengkit came up with his version that has no gaps but unproportional pieces. See images 4-5.
Size: 67 x 78 x 78 mm

Thank you to the following people for their assistance in helping collect the information on this page: Ilya Toporgilka, quisquidillius.

This puzzle can be found in collections of these members:

---

Found a mistake or something missing? Edit it yourself or contact the moderator.